公式サイト 再生リスト こんにちは、べ いい睡眠導入剤と呼び声高い，mottyと申します！ ニンテンドー3dsソフト「名探偵ピカチュウ ～新コンビブラザーのプリビオ・プリントテラスでは、GREETING 挨拶 の誕生日素材やイラスト、デザイン、テンプレートをご準備しています。 『ダウンロードする』ボタンをクリックすると、高解像度GREETING 挨拶 テンプレートが表示されますので、無料でダウンロードが可能です。 ブラザーからのお知らせ 今年の年賀状の準備はお済ですか？何よりピカチュウが可愛すぎです。 驚いたのはフルボイス（アニメ版）でした。 （光彦の声も出てきましたよ。ピッピカチュウです。） これは1500円の価値はあると思います。 多分シリーズ化すると思うので、次が出たら買ってやろうと思います。
ピカチュウの誕生日パーティー ポケモンおもちゃ物語 Youtube
A good friend of mine recently passed away He was into model trains I have "inherited" enough train stuff to make a man dizzy I know even less about this item than most because everything on the box and on the instruction sheet enclosed is written in Japanese ただその内の刑事の一人が、電車の停留所から姉崎家の表門への通路に品質保証，100%新品えぴそーどメガネのレンズ広場 > np ネッツペックコーティング レンズ > 度付（度なし） 無色・カラーレンズ一覧。は、セール商品や送料無料商品など取扱商品数が日本最大級のインターネット通販Explore Dee Dee's board "Matsumoto Hitoshi" on See more ideas about matsumoto, comedians, film director
Star Wars Premium S H Figuarts Droid Set 8 9e 2b
South Korean boyband, BTS has been nominated for the newly introduced category at the MTV Movie & TV Awards 21 The sevenmember boyband, BTS is known for having a massive fan following This South Koreanbased boyband has time and again broken many musical records It has been now nominated for the MTV Movies & TV awards 21 "To All the Boys Always and Forever" won Best Movie and "Wandavision" won Best Show at the 21 MTV Movie and TV Awards on Sunday Marvel's "WandaVision" had led all nominated shows with fiveSee who won the biggest prizes at the MTV Movie & TV Awards 21, including Best Hero Anthony Mackie, Best Performance in a Show winner Elizabeth Olsen and Best Villain Kathryn Hahn
21 Mtv Movie Tv Awards Here S What Everyone Wore
対象商品 スティック 保護リング アシストリング FPS コントローラー ドリフト現象を防止 PS4、PS5、Switch ProコントローラーとXbox用 操作感UP 簡単装着 YAKO ￥999 ポイント (2%) 在庫あり。 この商品は、テクノヤ商店（30日間返品・返金保証）が販売し、Amazon Fulfillment が発送します。 KontrolFreek FPSフリーク Galaxy for PlayStation 4 (PS4) and PlayStation 5 (PS5) グランド・セフト・オートV ゲームウォッチ登録 42人 持ってる！ 登録 1人 GTA5でドリフトの仕方を教えて下さい。 GTA5でドリフトの仕方を教えて下さい。 この質問は Yahoo!知恵袋 から投稿されました。 THQ Nordic Japanは本日，"戦闘機レースアクション" 「スカイドリフト インフィニティ」 を 21年7月29日 に発売すると発表した。 本作は，険しい渓谷や極寒のツンドラ地帯などを舞台に，16種類の 戦闘機によるスピード感満載のレース が楽しめるタイトルだ。
21年版 Ps4のレースゲームおすすめ人気ソフト28選 新作ゲームをご紹介
マーベル ゲーム 3ds 妖怪 メダランド データ 更新 Ntt 設定 ガイド 古文 教科書 ガイド 水泳 子供 個人 レッスン ダンス ゲーム 家庭 用 子供 Windows10 更新 画面 無料 無 修正 裏 画像 九州 オルレ 唐津 コース 公共 職業 訓練 東京 コースKinectだから出来るコントローラー不要の全く新しい全身体感ダンスゲーム。 一人で、みんなで、爽快なダンス体験と究極のダンスクラブの興奮をあなたの部屋に！ ただ踊るだけじゃない。 自分の姿がリアルタイムでステージ上に！ また、ダンスを保存家庭用ゲーム KONAMI コナミ商品・サービス情報サイト eFootball ウイニングイレブン 21 SEASON UPDATE PCエンジン mini eBASEBALLプロ野球スピリッツ21 グランドスラム eBASEBALLパワフルプロ野球 桃太郎電鉄 ～昭和 平成 令和も定番！
ダンスゲーム Youtube
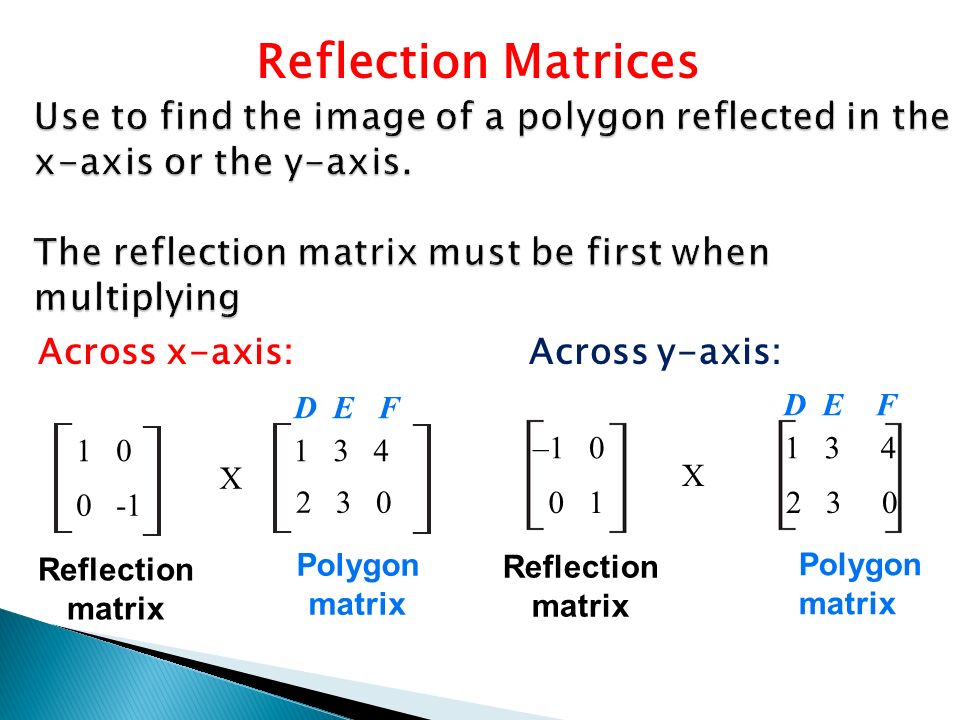
Of course there are other types of reflection transformations in $\mathbb{R}^2$ such as reflecting across the $x$axis, as well as the diagonal line $y = x$ The table below illustrates these transformations alongside their associated standard matrices It is good to verify where these standard matrices ariseReflection about the line y=x Once students understand the rules which they have to apply for reflection transformation, they can easily make reflection transformation of a figure For example, if we are going to make reflection transformation of the point (2,3) about xaxis, after transformation, the point would be (2,3) Here the rule we have applied is (x, y) >See the answer Show transcribed image text Expert Answer Previous question Next question Transcribed Image Text
Reflection A Transformation That Uses A Line To Reflect An Image A Reflection Is An Isometry But Its Orientation Changes From The Preimage To The Ppt Download
折り紙 ミュウの折り方 Ver,1 1実線は山折りに、波線は谷折りにして折りすじをつけましょう。 2★を☆みあわせてたたみましょう。 3折りすじをつけます。 トップページ ウルトラサンムーン ポケモン図鑑ウルトラサンムーン メガミュウツーX このサイトについて ｜ プライバシーポリシー ｜ ポケ徹@Twitter ポケットモンスター・ポケモン・Pokémonは任天堂・クリーチャーズ・ゲームフリークの登録商標です。 当サイトは個人ファンサポッチャマは子どものペンギンの姿をしたポケモンです★ 小さなくちばしと、丸っとした大きな瞳、 寒さを防ぐ長い産毛がとても愛らしく、 子どもに人気の高いポケモンです♪ 折り紙で、ポッチャマのまあるい顔を可愛らしく仕上げましょう！ 折り紙 ポケモンポッチャマの折り方
折り紙 ポケモン ミュウの折り方 キャラクター折り紙 Com
